Recall that holes exist in rational functions when the expressions numerator and denominator share a common factor. This value of x is still a domain restriction but it is represented as a Hole in the graph of.
Rational Function Holes Explanation And Examples
The graph of a rational function usually has vertical asymptotes where the denominator equals 0.

. Let y b for x a. So there is a hole at x a. -1 -2 left0 -dfrac12right and left3 dfrac25right.
This problem has been solved. If as x approaches some. X - a 0.
In Example 9 we see that the numerator of a rational function reveals the x -intercepts of the graph whereas the denominator reveals the vertical asymptotes of the graph. Up to 10 cash back This excluded value is usually referred to as hole in the rational function. This occurs when a common real factor shows up in the numerator and denominator.
The graph of a rational function sometimes has a hole. True or False The graph of a rational function sometimes has a hole. The only difference between the slant asymptote of the rational function and the rational function itself is that the rational function isnt defined at x 2To account for this I leave a nice big open circle at the point where x 2 showing that I know that this point is not actually included on the graph because of the zero in the denominator of the rational.
If a function is even or odd then half of the function can be. A rational function gx fx hx may sometimes have a hole in its graph. Decide whether the following statement is true or false.
When we do so we get. The solution is the x-value of the hole. Before putting the rational function into lowest terms factor the numerator and denominator.
This can sometimes save time in graphing rational functions. X a. The hole of the rational function is x 3.
Find the holes boldsymbolx-coordinate by equating this factor to boldsymbol0. If there is a common factor at both numerator and denominator there is a hole for the rational function. This means that the function has three holes as well.
Now simplify the rational function cross out the factor that is the. Cancel the factor in the expression for x2-5x fx x-r-46. Hence the graph of rational function sometimes have hole.
Important properties and elements of a rational functions graph. This occurs when there is a common factor in the. For example the rational function y 4 x 2 x 2 x 2 x has a hole at x 0.
Holes in the Graph of a Rational Function Sometimes the numerator and the denominator of a rational function are both zero at the same time. The domain of every rational function is the set of all real numbers. Rational Functions MATH 1330 Precalculus 229 Recall from Section 12 that an even function is symmetric with respect to the y-axis and an odd function is symmetric with respect to the origin.
Removable discontinuities occur when gxand hxshare a common factor. Students also viewed these Mathematics questions True or False The graph of a rational function sometimes intersects an. A rational function is a quotient of two functions.
As with polynomials factors of the numerator may have integer powers greater than one. For a rational function R if the degree of the numerator is less than the degree of the denominator then R is ____. If there is the same factor in the numerator and denominator there is a hole.
Find the coordinates of the hole in the graph of the rational function fx 7-2 22-2-6 A B. Explain why the graph of a rational function cannot have both a Consider a hypothetical computer. The graph of a rational function sometimes has a hole.
I The graph of. Learn how to graph a rational function. Let x - a be the common factor found at both numerator and denominator.
And theres no assemble at X equals who So the graph of the function out of excellent have a whole X equals two who that the value of the country is not keeping. It shows that this point is not present on the graph of the rational function. Please note that the graphs of the rational functions satisfy the vertical line test.
Decide whether the following statement is true or false. Consider a rational function in the form of p q where q 0 has some factors which are easily divisible by the numerator value. So the hole will appear on the graph at the point a b.
It is possible to have a rational. Now we have to make x - a equal to zero. In calculus these holes are called removable discontinuities.
However the graph of a rational function will have a hole when a value of x causes both the numerator and the denominator to equal 0. Set this factor equal to zero and solve. Which asymptote will never intersect the graph of a rational function.
The graph does not have a hole. Holes - Sometimes graphs of Rational Functions can contain a Holes. Look at the degrees of the polynomials involved Question.
Plot the holes of the rational function. If false give a counterexample. The graph of the function f x3x4-x35x2-2x-7 will behave like the graph of ______ for larger values of x.
As a Vertical Asymptote. In this case the zero in the denominator need not cause the graph to have a vertical asymptote. True or False The graph of a rational function sometimes interscets.
A- Horizontal Asymptote B- Vertical Asymptote C- Oblique Asymptote. The graph does not have a hole. The graph shown above contains three unfilled points at.
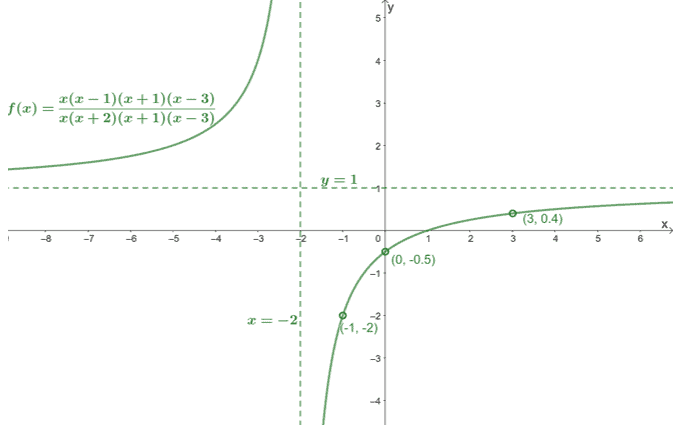
I The graph of a rational function can never have more than one horizontal asymptote If true explain why. The graph shows that the graph has discontinuities at the hole and its vertical asymptote at x -2. True or False The graph of a rational function sometimes has a hole.
Every rational function has to have at least one asymptote. Graphing Rational Functions with Holes. Find the coordinates of the hole in the graph of the rational function fx 7-2 22-2-6 A B.
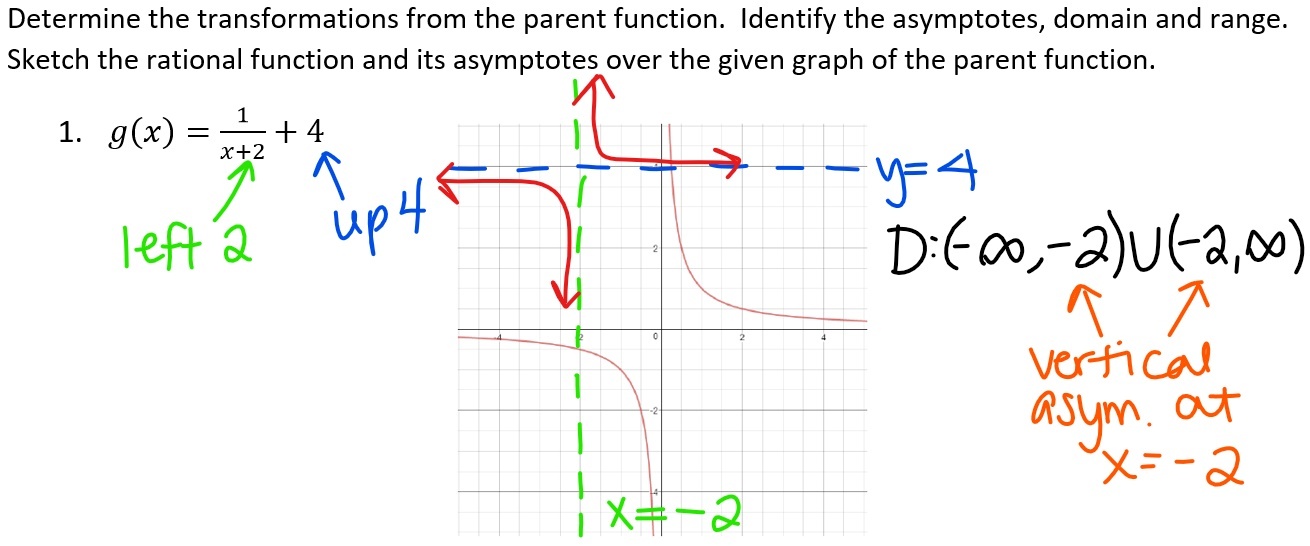
It is possible to have holes in the graph of a rational function. To graph a rational function we first find the vertical and horizontal or slant asymptotes and the x and y-interc. Fortunately the effect on the shape of the graph at those.

Understanding Graph Of This Rational Function Mathematics Stack Exchange
5 4 Rational Functions Mathematics Quizizz

Alex S Roller Coaster Creating A Polynomial Function Polynomial Functions Polynomials Rational Function
0 Comments